Basic Statistics

There are primarily two branches in which basic statistics are studied:

Descriptive Statistics

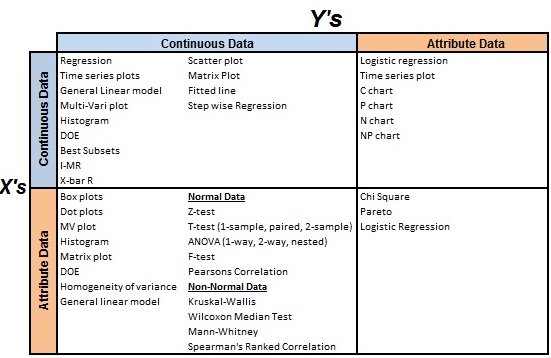
Applied to describe the data using numbers, charts, and graphs. Terms such as mean, median, mode, variance, standard deviation are values that summarize data. Descriptive statistics describe the entire group for which the numbers were obtained. These are the actual values for the entire group.

Inferential Statistics

Uses sample statistics to infer relationships of the population parameters. This is most often done in the Analyze and Improve phases using hypothesis testing, correlation analysis, regression analysis, and design of experiments (DOE).

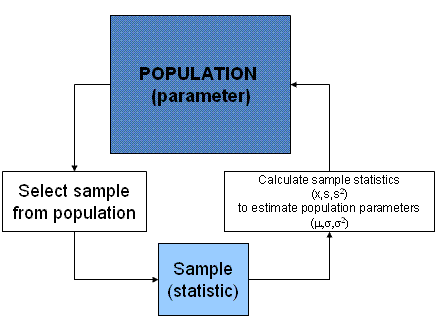
It is rarely possible to analyze the entire population (such as the average weight of all sharks in the ocean). For this reason a sampling strategy is applied. The analysis of the sample is used to apply inferences to the entire population. The values may or may not be the same values for the entire population so they are applied with a confidence interval.

A comparison of means, variance, or proportions is initiated with a hypothesis statement about a population or populations. The sample statistics are studied to determine, with a certain level of confidence and power, that the hypothesis (null hypothesis) is to be proven false or not false but not necessarily true. See Hypothesis Testing for more information.

The links below cover other topics within basic statistics:

Graphical Summaries

Histograms

Box Plots

Stem and Leaf Plot

Hypothesis Testing

Hypothesis Testing

Normality Assumption

Critical Values

Central Limit Theorem

Analysis of Variance (ANOVA)

Proportions

T-Tests

Chi-Square

"Defect" Metrics

DPU - Defects Per Unit

DPO - Defects Per Opportunity

DPMO - Defects Per Million Opportunities

Process Yield Metrics

FY - Final Yield

TPY - Throughput Yield

RTY - Rolled Throughput Yield

NY - Normalized Yield

The meaning and formula around sigma scores

Z-score

Yield to Sigma Relationships

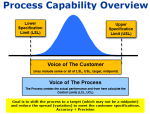
Process Capability Indices

Pp

Ppk

Cp

Cpk

Cpm

Cm

Cmk

Distributions:

Discrete Distributions

Continuous Distributions

Shape of the Distribution:

Kurtosis

Skewness

Other topics

SPC - Statistical Process Control

Hypothesis Tests Flowcharts

Hypothesis Testing

Alpha and Beta Risks (Type I Error and Type II Error)

What are Critical Values?

Power and Sample Size

Comparing Samples vs. Population

Short Term versus Long Term sampling

Data Classification

Data Sampling

Multi-variate Analysis

P-Value

Confidence Intervals

Margin of Error

Correlation

Regression

Covariance

Measures of Central Tendency: Mean, Median, Mode

Measures of Dispersion: Range, Standard Dev, Variance, Coefficient of Variation

Probability Density Function

Cumulative Distribution Function

Normal Distribution

Degrees of Freedom

Box-Cox Transformation

ShareFacebookXWhatsApp

Basic Statistics - Distributions

DISCRETE DISTRIBUTIONS:

1. Binomial Distribution
2. Poisson Distribution
3. Hypergeometric Distribution
   

CONTINUOUS DISTRIBUTIONS:

A few other continuous distributions (but are not covered in this website) are Beta, Cauchy, Gamma, Lognormal, Weibull, Double Exponential, Power Normal, Bivariate Normal, Power Log Normal, Triangular, and Tukey-Lambda.

Statistics Evaluation Tools

Six-Sigma-Material.com

Return to the MEASURE phase

Return to the ANALYZE phase

Templates, Tables, and Calculators

Find a career in Six Sigma - active job postings

Return to the Six-Sigma-Material Home Page